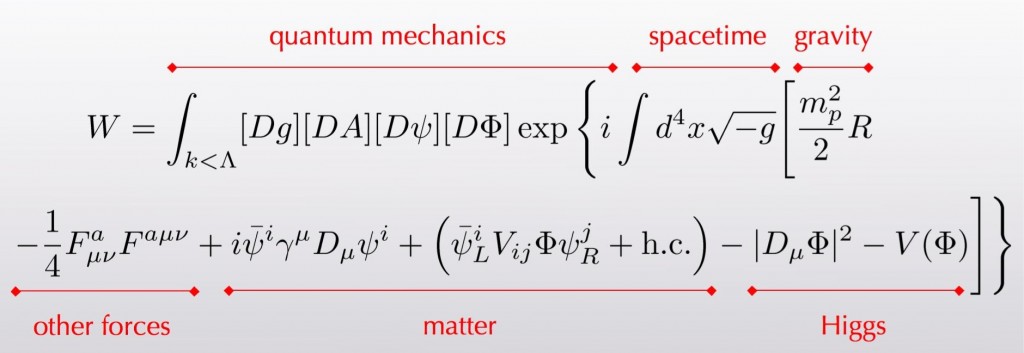
Longtime readers know I feel strongly that it should be more widely appreciated that the laws underlying the physics of everyday life are completely understood. (If you need more convincing: here, here, here.) For purposes of one of my talks next week in Oxford, I thought it would be useful to actually summarize those laws on a slide. Here’s the most compact way I could think to do it, while retaining some useful information. (As Feynman has pointed out, every equation in the world can be written U=0, for some definition of U — but it might not be useful.) Click to embiggen.
This is the amplitude to undergo a transition from one configuration to another in the path-integral formalism of quantum mechanics, within the framework of quantum field theory, with field content and dynamics described by general relativity (for gravity) and the Standard Model of particle physics (for everything else). The notations in red are just meant to be suggestive, don’t take them too seriously. But we see all the parts of known microscopic physics there — all the particles and forces. (We don’t understand the full theory of quantum gravity, but we understand it perfectly well at the everyday level. An ultraviolet cutoff fixes problems with renormalization.) No experiment ever done here on Earth has contradicted this model.
Obviously, observations of the rest of the universe, in particular those that imply the existence of dark matter, can’t be accounted for in this model. Equally obviously, there’s plenty we don’t know about physics beyond the everyday, e.g. at the origin of the universe. Most blindingly obvious of all, the fact that we know the underlying microphysics doesn’t say anything at all about our knowledge of all the complex collective phenomena of macroscopic reality, so please don’t be the tiresome person who complains that I’m suggesting otherwise.
As physics advances forward, we will add to our understanding. This simple equation, however, will continue to be accurate in the everyday realm. It’s not like the Steady State cosmology or the plum-pudding model of the atom or the Ptolemaic solar system, which were simply incorrect and have been replaced. This theory is correct in its domain of applicability. It’s one of the proudest intellectual accomplishments we human beings can boast of.
Many people resist the implication that this theory is good enough to account for the physics underlying phenomena such as life, or consciousness. They could, in principle, be right, of course; but the only way that could happen is if our understanding of quantum field theory is completely wrong. When deciding between “life and the brain are complicated and I don’t understand them yet, but if we work harder I think we can do it” and “I understand consciousness well enough to conclude that it can’t possibly be explained within known physics,” it’s an easy choice for me.
Let me know if I’ve made any typos here, or have gone too far in trying to make things compact. For instance, can I get away without putting a “trace” around the gauge field kinetic term? I don’t want a notational shortcut to undermine my argument and leave the audience believing in God.
Is the cosmological constant hidden in the Higgs potential?
“We don’t understand the full theory of quantum gravity, but we understand it perfectly well at the everyday level.”
You understand gravity? Great, please explain to me what physically pulls the atoms of my body and the Earth together? What object connects the atoms, mediating the force of pull? Please answer.
I posted this on Facebook as well but figured I’d post it here to. If what you say is true – that we absolutely fully understand the physics of everyday life – then why did we even bother with that conference 18 months ago? I can’t imagine it was just for the purpose of a nice boat ride in the North Sea.
Quantum field theory obviously makes very accurate predictions, but that doesn’t necessarily mean it matches reality. A large part of my everyday experience involves my mind. I am not aware of any full explanation of the mind in physical terms yet.
Plus I think it’s a little disingenuous to make the claim that quantum field theory can fully explain everyday life given that we don’t fully understand emergence yet nor to we fully understand complex systems.
The only typo I see is in the 4th paragraph: …were simply incorrect and have been replace_d_.
BTW, I’m currently reading “The Particle At The End Of The Universe” and enjoying it as well as this post. Thanks, Sean.
Robert– Yes, but most of us go through our everyday lives unaffected by the cosmological constant.
Mike– Yes, I do understand gravity, I even wrote a whole textbook about it. Check it out!
Ian– It’s the physics underlying everyday life. All of the words mean something. (See above about tiresomeness.)
Sean — I agree (of course).
Since it’s an effective theory I just thought that it would be best to include the lowest dimensional operator in the gravity sector.
OK, I guess I really don’t understand what you’re saying, then. Because I could just as easily say that the equation does not “explain” entanglement (if it did, I’d be out of a job) and you’d likely counter by saying it has nothing to do with everyday experience. But if you’re talking about the physics underlying everyday experience then how do we even know what that is to begin with if we don’t understand emergence and complexity?
Beautiful work, Sean! I’ll need to do some googling to figure out what the individual terms represent but thank you for the map explaining the big pieces of it.
Sean, are you trying to be provocative on purpose? 🙂
Leaving aside all the philosophical stuff about consciousness, God, etc., the gravitational sector of that equation is all but understood. Care to give a definition for the path-integral measure [Dg]? Do you sum over all possible spacetime topologies or not? What about the divergent loop-corrections for gravitons? And no, one can’t even get the classical limit (i.e. general relativity) from the above equation.
So not even everyday life, sorry.
There are also some missing pieces in the matter sector, like the proton mass prediction, etc. But those are probably just technical points.
Best, 🙂
Marko
The equation predicts any observable phenomenon associated with what we call “entanglement.” That’s all one can ask for: a model that makes accurate predictions.
If we discover our minds each have access to a pinpoint wormhole, does that mean our understanding of quantum theory is wrong, or our contemplation of what constitutes everyday experience is flawed?
That’s nicely succinct. I do think you can get away with writing the trace in the gauge kinetic term as an implied sum over Lie algebra components, as you’ve done, since the Lie algebra basis generators are usually chosen such that the Killing form is +1 down the diagonal. There might be some trickiness for neutrinos, if they’re not purely Dirac fermions or they have Majorana masses. You should also probably include the cosmological constant. And there will probably need to be some kind of inflaton multiplying the scalar curvature, but there’s not consensus on how that works yet. And it is important to emphasize that this description doesn’t work well for quantum gravity; so as marvelous as it is, it’s still not ready to be chiseled into stone yet. But for describing the physics behind everyday life, it’s sufficient, and probably overkill!
“Mike– Yes, I do understand gravity, I even wrote a whole textbook about it. Check it out!”
Excellent, but I was hoping you could just help me to visualize the process right now, in THIS thread, because I am not convinced.
I read through a couple of your articles and on the one about explaining how Higgs Bosons work I found this:
“Now of course you should probably try to explain why fog would give mass to something. That’s left as an exercise for the reader.”
Is this still your position on the Theory? How can a fog mediate the force of PULL between objects? Isn’t that what we are talking about with gravity anyway, the phenomena of tension between all objects?
I wonder whether it is fair to say that the laws underlying the physics of everyday life are understood if those laws cannot predict the outcome of all given events of everyday life in the same way they can predict the outcome of any given experiment designed to test them. It seems like the word understood is being used in 2 different ways. Is it a hypothesis, or is it a fact, to say that all of the events of everyday life will conform to the same underlying laws that we have tested experimentally thus far? Does “understood” imply empirically tested, or can a hypothesis be enough?
One thing is for sure we are part of a system and the system would be different without us
I actually try to be both provocative and irrationally simultaneously ignorant and arrogant to try to get under the skin of people I find to be pompous asses.
There are two, and only two, possibilities when it comes to understanding the physics of everyday life. The first is that we know enough to completely describe the physics of everyday life. The second is that we will never know enough to “completely” understand the physics of everyday life because it is impossible. Just as it would be impossible to know all information about the universe. I could understand both stances on the topic, but agree most with what Sean is saying. I would prefer to spend my time arguing about how FUC**NG AMAZING this is and if anyone understands how truly important it is; http://www.sciencemag.org/content/339/6115/52
Neat stuff! And i especially like the last tongue in cheeker: “I don’t want a notational shortcut to undermine my argument and leave the audience believing in God.
And, since you asked: some typos/edits:
“For purposes of one of my talks”… Just “for one of my talks…” or “for the purposes of…” are better.
“embiggen” should probably be “enbiggen?” We don’t say “emlarge,” so, it should probably stay relative to known good semantics. Just like quantum does not replace relativity, so fun words are more appreciated and need to grow from their predecessors and kin.
“Equally obviously” should be “equally obvious”
“As physics advances forward”… redundant: “As physics advances” is right.
In the infinite universes theory there could be an infinite number of exact Seans, or any of us, with the exact particle make up, why in reality is there only one of us? There is absolutely no possibility of mathematics or physics or any of the sciences proving the possibility of a higher state of existence, sorry, it simply can’t be done, it’s far, far beyond anything in this universe. If physics can prove the existence of a Love that is substantive, like a solid, such as rock, then it could, but it can’t, it simply can’t, no way, no how, never, never, sorry and I know of what I say.
moonside0
I like that as a start to how to approach the real question.
To me there is no such thing as a “complete understanding,” we simply know more than we used to but a “complete understanding” is a complete fiction. If you keep asking further questions you run out of answers almost immediately.
Why anything at all? Why three spatial dimensions and one time dimension? Why curved spacetime? Why Born rule? Why Pauli exclusion principle? Why these symmetries? Why those masses? Why three families? etc, etc…
In fact I would say the opposite is true – in reality we don’t understand a damn thing about the laws of physics. All these laws amount to is an efficient description – not explanation – of the patterns we’ve found by trial and error.
I’m going to take a crack at describing what I think is meant by “the laws underlying the physics of everyday life are completely understood.” This is generally for my own benefit – I’d like to share this post but I want to make sure I can explain it in my own words. Sean, if you read this comment and I’m out in left field please straighten me out.
Physics of everyday life means we’re talking about ordinary run-of-the-mill matter and fields in ordinary run-of-the-mill quantities and energies. Specifically we’re not talking about black holes, firewalls or exotic particles living brief lives in an LHC experiment. We’re talking about protons, neutrons, electrons, atoms, molecules, EM fields, electric current, yadda, yadda, yadda.
That the rules are completely understood means exactly that (and not more). The existing theories provide rules describing how this matter and energy interact. Given a state of a system the rules predict how that state will evolve with time. When these rules are tested against physical reality they are always correct. There is no experimental reason to doubt that these rules really do describe how the run-of-the-mill stuff of ordinary life actually behave.
That’s pretty much it. So what does it really mean? Well one thing it doesn’t mean is that just because we understand the rules we can predict how every system will behave. A system may be composed of so many parts in such a complex arrangement that we simply don’t have the physical capability to work out all the details. But, under the covers, the interactions of all those many parts is governed by the known physical rules and nothing else. Even though a system is too complex for us to model there is nothing happening inside that isn’t described by the known rules of the physics. For example, we may not be able to predict right now exactly how a protein will fold up but when we are able to work it out we won’t need to invent any new rules of physics to explain it.
Also, there is no room left for magic or the supernatural. A magical event would entail observing something in the ordinary physical world behaving in a way not predicted by the rules of physics. No observation of the physical world has ever encountered such an event. There is nothing we can imagine doing to objects in the everyday world that isn’t covered by the rules of physics and hasn’t been experimentally verified.
Thus we can legitimately claim to completely understand the physical rules of everyday life.
“But if you’re talking about the physics underlying everyday experience then how do we even know what that is to begin with if we don’t understand emergence and complexity?”
Because we don’t need to understand every move a chess grandmaster makes to convince ourselves we understand the basic underlying rules of chess, to take a well known analogy.
I share sean’s position that it’s tiresome having to defend a point that shouldn’t be controversial. It’s like saying the rules underlying chemistry are well known and complete, which they are, then someone goes “oh yeah? Well derive the spectrum of all atoms in the periodic table from first principles. Until then you can’t say quantum mechanics explains chemistry”. Yet no chemist would argue against the fact that quantum mechanics provides a complete description of all chemistry.
MarcS– I think that’s a fair way of putting it. I’ve elaborated at length on what is meant by the claim in the various links included in the post, but the more ways of saying it the better.
Thanks Sean..
There’s another such equation, by the way, that was displayed by Neil Turok during his lecture (What Banged?) (available on Youtube).
Are there any basic differences between the two ?
MarcS and Sean,
It seems like what you’re saying is that we completely understand the constraints on what the known universe can present to us. Any experience we have in everyday life will not fall outside of those constraints. Within those constraints, things can happen that we cannot predict or fully understand at present, but those events are limited by the above stated constraints that we have discerned. No more surprises.
Seems fair if that is what you are saying.
So is it time to focus mainly on the operations that still elude us within those constraints? Seems like that is where our energy and money ought to go.